
Since the p-value is less than our chosen significance level α = 0.05, we can reject the null hypothesis, and conclude that there is an association between class rank and whether or not students live on-campus.īased on the results, we can state the following:

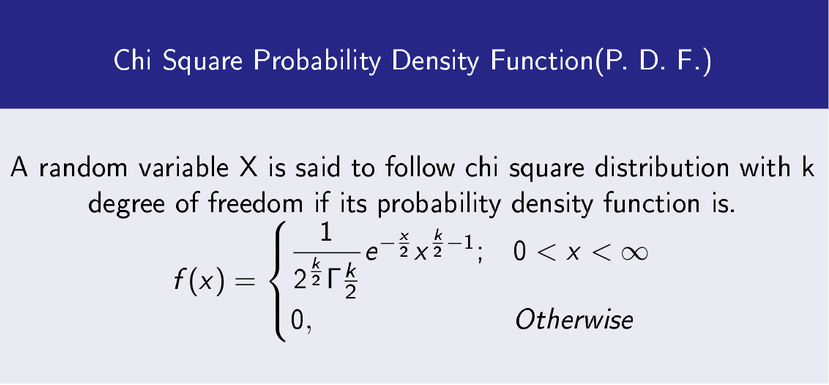
Because the crosstabulation is a 2x2 table, the degrees of freedom (df) for the test statistic is $$ df = (R - 1)*(C - 1) = (2 - 1)*(2 - 1) = 1 $$.The value of the test statistic is 138.926.We can confirm this computation with the results in the table labeled Statistics for Table of RankUpperUnder by LiveOnCampus: Computation of the expected cell counts and residuals (observed minus expected) for the crosstabulation of class rank by living on campus. We can use JMP to perform the standard deviation test by selecting the Shelf Life. Thus there is insufficient evidence to conclude the variance exceeds 225 or equivalently the standard deviation exceeds 15. With the Expected Count values shown, we can confirm that all cells have an expected value greater than 5. The root mean square chiral index CHI of a d-dimensional set of n points was defined12 as the sum of the n squared distances between the vertices of the set. 641 of your text we find the following upper and lower bound on the p-value to be. If you included the EXPECTED and DEVIATION options in your syntax, you should see the following: The first table in the output is the crosstabulation. TABLE RankUpperUnder*LiveOnCampus / CHISQ EXPECTED DEVIATION NOROW NOCOL NOPERCENT Suppose that we want to test the association between class rank and living on campus using a Chi-Square Test of Independence (using α = 0.05). The proportion of upperclassmen who live on campus is 5.6%, or 9/161.The proportion of upperclassmen who live off campus is 94.4%, or 152/161.The proportion of underclassmen who live on campus is 65.2%, or 148/227.I am to estimate the chi square between two samples of size N and M, where in general N << M (e.g. Identify the degrees of freedom for the test you are doing and for your data.
If you set 0.05 for your test, then find the column for 0.05. Each column in the table lists values for different alpha levels. The data entry and analysis are identical to that used for tests on a.
#Chi square jmp how to
The proportion of underclassmen who live off campus is 34.8%, or 79/227. Chi square test between samples with very different size. The steps below describe how to use a typical Chi-square table. JMP-IN will do a chi-squared goodness-of-fit test in the Distribution of Y Platform.Recall that the column percentages of the crosstab appeared to indicate that upperclassmen were less likely than underclassmen to live on campus: Let's continue the row and column percentage example from the Crosstabs tutorial, which described the relationship between the variables RankUpperUnder (upperclassman/underclassman) and LivesOnCampus (lives on campus/lives off-campus).


 0 kommentar(er)
0 kommentar(er)
